You are here : Control System Design - Index | Book Contents | Appendix C | Section C.2

C. Results from Analytic Function Theory

C.2 Independence of Path

Consider functions of two independent variables, $x$ and $y$. (The reader can think of $x$ as the real axis and $y$ as the imaginary axis.)

Let $P(x,y)$ and $Q(x,y)$ be two functions of $x$ and $y$, continuous in some domain $D$. Say we have a curve $C$ in $D$, described by the parametric equations


\begin{displaymath}x=f_1(t),\hspace{20mm}y=f_2(t) \end{displaymath} (C.2.1)

We can then define the following line integrals along the path $C$from point $A$ to point $B$ inside $D$.


$\displaystyle \int_A^BP(x,y)dx$ $\textstyle =$ $\displaystyle \int_{t_1}^{t_2}P(f_1(t),f_2(t))\frac{df_1(t)}{dt}dt$ (C.2.2)
$\displaystyle \int_A^BQ(x,y)dy$ $\textstyle =$ $\displaystyle \int_{t_1}^{t_2}Q(f_1(t),f_2(t))\frac{df_2(t)}{dt}dt$ (C.2.3)

Definition C.1  The line integral $\int P dx +Q dy$ is said to be independent of the path in $D$ if, for every pair of points $A$ and $B$ in $D$, the value of the integral is independent of the path followed from $A$ to $B$.

$\Box \Box \Box $

We then have the following result.

Theorem C.1  If $\int P dx +Q dy$ is independent of the path in $D$, then there exists a function $F(x,y)$ in $D$ such that

\begin{displaymath} \frac{\partial F}{\partial x}=P(x,y); \hspace{20mm}\frac{\partial F}{\partial y}=Q(x,y) \end{displaymath} (C.2.4)

hold throughout $D$. Conversely, if a function $F(x,y)$ can be found such that (C.2.4) hold, then $\int P dx +Q dy$is independent of the path.

Proof

Suppose that the integral is independent of the path in $D$. Then, choose a point $(x_0,y_0)$ in $D$ and let $F(x,y)$ be defined as follows


\begin{displaymath}F(x,y)=\int_{x_0,y_0}^{x,y}P dx +Q dy \end{displaymath} (C.2.5)

where the integral is taken on an arbitrary path in $D$joining $(x_0,y_0)$ and $(x,y)$. Because the integral is independent of the path, the integral does indeed depend only on $(x,y)$ and defines the function $F(x,y)$. It remains to establish (C.2.4).


Figure C.1: Integration path
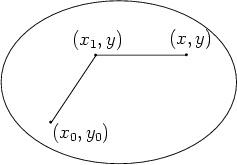\begin{figure} \begin{center} \leavevmode \begin{picture} (0,0)% \epsfig{file=... ...lt}{\mddefault}{\updefault}$(x_0,y_0)$ }}} \end{picture}\end{center}\end{figure}

For a particular $(x,y)$ in $D$, choose $(x_1,y)$ so that $x_1\neq x$ and so that the line segment from $(x_1,y)$ to $(x,y)$ in $D$is as shown in Figure C.1. Because of independence of the path,


\begin{displaymath} F(x,y)=\int_{x_0,y_0}^{x_1,y}(P dx +Q dy)+ \int_{x_1,y}^{x,y}(P dx +Q dy) \end{displaymath} (C.2.6)

We think of $x_1$ and $y$ as being fixed while $(x,y)$ may vary along the horizontal line segment. Thus $F(x,y)$ is being considered as function of $x$. The first integral on the right-hand side of (C.2.6) is then independent of $x$.

Hence, for fixed $y$, we can write


\begin{displaymath}F(x,y)=\mbox{constant} +\int_{x_1}^{x}P(x,y) dx \end{displaymath} (C.2.7)

The fundamental theorem of Calculus now gives


\begin{displaymath}\frac{\partial F }{\partial x }=P(x,y) \end{displaymath} (C.2.8)

A similar argument shows that


\begin{displaymath}\frac{\partial F }{\partial y}=Q(x,y) \end{displaymath} (C.2.9)

Conversely, let (C.2.4) hold for some $F$. Then, with $t$ as a parameter,


$\displaystyle F(x,y)=\int_{x_1,y_1}^{x_2,y_2}P dx +Q dy$ $\textstyle =$ $\displaystyle \int_{t_1}^{t_2} \left ( \frac{\partial F }{\partial x }\frac{dx}{dt}+\frac{\partial F }{\partial y }\frac{dy}{dt} \right) dt$ (C.2.10)
  $\textstyle =$ $\displaystyle \int_{t_1}^{t_2}\frac{\partial F }{\partial t }dt$ (C.2.11)
  $\textstyle =$ $\displaystyle F(x_2,y_2)-F(x_1,y_1)$ (C.2.12)

$\Box \Box \Box $

Theorem C.2  If the integral $\int P dx +Q dy$ is independent of the path in $D$, then


\begin{displaymath} \oint P dx +Q dy =0 \end{displaymath} (C.2.13)

on every closed path in $D$. Conversely if (C.2.13) holds for every simple closed path in $D$, then $\int P dx +Q dy$ is independent of the path in $D$.


Figure C.2: Integration path
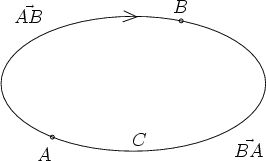\begin{figure} \begin{center} \leavevmode \begin{picture} (0,0)% \epsfig{file=... ...ult}{\mddefault}{\updefault}$\vec{AB}$ }}} \end{picture}\end{center}\end{figure}

Proof

Suppose that the integral is independent of the path. Let $C$ be a simple closed path in $D$, and divide $C$ into arcs $\vec{AB}$ and $\vec{BA}$ as in Figure C.2.


$\displaystyle \oint_C (Pdx+ Q dy)$ $\textstyle =$ $\displaystyle \int_{AB}Pdx+Qdy+\int_{BA}Pdx+Qdy$ (C.2.14)
  $\textstyle =$ $\displaystyle \int_{AB}Pdx+Qdy-\int_{AB}Pdx+Qdy$ (C.2.15)

The converse result is established by reversing the above argument.

$\Box \Box \Box $

Theorem C.3  If $P(x,y)$ and $Q(x,y)$ have continuous partial derivatives in $D$ and $\int P dx +Q dy$ is independent of the path in $D$, then


\begin{displaymath} \frac{\partial P}{\partial y}=\frac{\partial Q}{\partial x} \hspace{10mm}\mbox{in $D$ } \end{displaymath} (C.2.16)

Proof

By Theorem C.1, there exists a function $F$ such that (C.2.4) holds. Equation (C.2.16) follows by partial differentiation.

$\Box \Box \Box $

Actually, we will be particularly interested in the converse to Theorem C.3. However, this holds under slightly more restrictive assumptions, namely a simply connected domain.