You are here : Control System Design - Index | Book Contents | Appendix C | Section C.6

C. Results from Analytic Function Theory


C.6 Analytic Functions

Definition C.2  A function $f(z)$ is said to be analytic in a domain $D$ if $f$ has a continuous derivative in $D$.

$\Box \Box \Box $

Theorem C.6   If $w=f(z)=u+jv$ is analytic in $D$, then $u$ and $v$ have continuous partial derivatives satisfying the Cauchy-Riemman conditions.


\begin{displaymath}\frac{\partial u}{\partial x}=\frac{\partial v }{\partial y};... ...m}\frac{\partial u}{\partial y}=-\frac{\partial v}{\partial x} \end{displaymath} (C.6.1)

Furthermore


\begin{displaymath}\frac{\partial w}{\partial z}=\frac{\partial u}{\partial x}+j... ...= \frac{\partial v}{\partial y}-j\frac{\partial u}{\partial y} \end{displaymath} (C.6.2)

Proof

Let $z_0$ be a fixed point in $D$ and let $\Delta\omega=f(z_0+\Delta z)-f(z_0)$. Because $f$ is analytic, we have


\begin{displaymath}\Delta\omega=\gamma \Delta z+\epsilon \Delta z; \hspace{10mm}\gamma\stackrel{\rm\triangle}{=}f'(z_0) \end{displaymath} (C.6.3)

where $\gamma=a+jb$ and $\epsilon$ goes to zero as $\vert z_0\vert$ goes to zero. Then


\begin{displaymath}\Delta u +j\Delta v=(a+jb)(\Delta x +j\Delta y)+ (\epsilon_1+j\epsilon_2)(\Delta x +j\Delta y) \end{displaymath} (C.6.4)

So



$\displaystyle \Delta u$ $\textstyle =$ $\displaystyle a\Delta x-b\Delta y+\epsilon_1\Delta x-\epsilon_2\Delta y$ (C.6.5)
$\displaystyle \Delta v$ $\textstyle =$ $\displaystyle b\Delta x+a\Delta y+\epsilon_2\Delta x+\epsilon_1\Delta y$ (C.6.6)

Thus, in the limit, we can write


\begin{displaymath}du=adx-bdy; \hspace{10mm}dv=bdx-ady \end{displaymath} (C.6.7)

or


\begin{displaymath}\frac{\partial u }{\partial x}=a=-\frac{\partial v }{\partial... ...ac{\partial u}{\partial y}=-b=-\frac{\partial v }{\partial x } \end{displaymath} (C.6.8)

$\Box \Box \Box $

Actually, most functions that we will encounter will be analytic, provided the derivative exists. We illustrate this with some examples.

Example C.1   Consider the function $f(z)=z^2$. Then


\begin{displaymath}f(z)=(x+jy)^2=x^2-y^2+j(2xy)=u+j v \end{displaymath} (C.6.9)

The partial derivatives are


\begin{displaymath}\frac{\partial u}{\partial x}=2x;\hspace{10mm} \frac{\partial... ...\partial y}=-2y;\hspace{10mm} \frac{\partial v}{\partial y}=2x \end{displaymath} (C.6.10)

Hence, the function is clearly analytic.

Example C.2   Consider $f(z)=\vert z\vert$ .

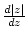This function is not analytic, because $d\vert z\vert$ is a real quantity and, hence, $\frac{d\vert z\vert}{dz} $ will depend on the direction of $z$.

Example C.3   Consider a rational function of the form:


\begin{displaymath} W(z)=K\frac{(z-\beta_1)(z-\beta_2)\cdots (z-\beta_m)} {(z-\alpha_1)(z-\alpha_2)\cdots (z-\alpha_n)}=\frac{N(z)}{D(z)} \end{displaymath} (C.6.11)


\begin{displaymath}\frac{\partial W}{\partial z}=\frac{1}{D^2(z)} \left[ D(z)\fr... ...(z)}{\partial z}-N(z) \frac{\partial D(z)}{\partial z} \right] \end{displaymath} (C.6.12)

These derivatives clearly exist, save when $D=0$, that is at the poles of $W(z)$.

Example C.4   Consider the same function $W(z)$ defined in (C.6.11). Then


\begin{displaymath}\frac{\partial \ln (W)}{\partial z}=\frac{1}{N(z)D(z)} \left[... ...)}{\partial z}- \frac{1}{D(z)}\frac{\partial D(z)}{\partial z} \end{displaymath} (C.6.13)

Hence, $\ln (W(z))$ is analytic, save at the poles and zeros of $W(z)$.