You are here : Control System Design - Index | Book Contents | Appendix D | Section D.1

D. Properties of Continuous-Time Riccati Equations


D.1 Solutions of the CTDRE

The following lemma gives a useful alternative expression for $\ensuremath{\mathbf{P}} (t)$.

Lemma D.1 The solution, $\ensuremath{\mathbf{P}} (t)$, to the CTDRE (D.0.1), can be expressed as


\begin{displaymath} \ensuremath{\mathbf{P}} (t)=\ensuremath{\mathbf{N}} (t)[\ensuremath{\mathbf{M}} (t)]^{-1} \end{displaymath} (D.1.1)

where $\ensuremath{\mathbf{M}} (t)\in \mathbb{R} ^{n\times n}$ and $\ensuremath{\mathbf{N}} (t)\in \mathbb{R} ^{n\times n}$ satisfy the following equation:

\begin{displaymath} \frac{d}{dt} \begin{bmatrix} \ensuremath{\mathbf{M}} (t)\\... ...th{\mathbf{M}} (t)\\ \ensuremath{\mathbf{N}} (t) \end{bmatrix}\end{displaymath} (D.1.2)

subject to


\begin{displaymath} \ensuremath{\mathbf{N}} (t_f)[\ensuremath{\mathbf{M}} (t_f)]^{-1}=\ensuremath{\boldsymbol{\Psi}} _f \end{displaymath} (D.1.3)

Proof

We show that $\ensuremath{\mathbf{P}} (t)$, as defined above, satisfies the CTDRE. We first have that


\begin{displaymath} \frac{d\ensuremath{\mathbf{P}} (t)}{dt}=\frac{d\ensuremath{... ...\mathbf{N}} (t)\frac{d[\ensuremath{\mathbf{M}} (t)]^{-1}}{dt} \end{displaymath} (D.1.4)

The derivative of $[\ensuremath{\mathbf{M}} (t)]^{-1}$ can be computed by noting that $\ensuremath{\mathbf{M}} (t)[\ensuremath{\mathbf{M}} (t)]^{-1}=\ensuremath{\mathbf{I}} $; then


\begin{displaymath}\frac{d\ensuremath{\mathbf{I}} }{dt}=\ensuremath{\mathbf{0}} ... ...\mathbf{M}} (t) \frac{d[\ensuremath{\mathbf{M}} (t)]^{-1}}{dt} \end{displaymath} (D.1.5)

from which we obtain


\begin{displaymath}\frac{d[\ensuremath{\mathbf{M}} (t)]^{-1}}{dt}=-[\ensuremath{... ...uremath{\mathbf{M}} (t)}{dt}[\ensuremath{\mathbf{M}} (t)]^{-1} \end{displaymath} (D.1.6)

Thus, Equation (D.1.4) can be used with (D.1.2) to yield


\begin{displaymath} \begin{split} - \frac{d\ensuremath{\mathbf{P}} (t)}{dt}&=\e... ...h{\mathbf{N}} (t)[\ensuremath{\mathbf{M}} (t)]^{-1} \end{split}\end{displaymath} (D.1.7)

which shows that $\ensuremath{\mathbf{P}} (t)$ also satisfies (D.0.1), upon using (D.1.1).

The matrix on the right-hand side of (D.1.2), namely,


\begin{displaymath} \ensuremath{\mathbf{H}} =\begin{bmatrix} \ensuremath{\math... ...space{20mm}\ensuremath{\mathbf{H}}\in\mathbb{R} ^{2n\times 2n} \end{displaymath} (D.1.8)

is called the Hamiltonian matrix associated with this problem.

Next, note that (D.0.1) can be expressed in compact form as


\begin{displaymath} \begin{bmatrix} -\ensuremath{\mathbf{P}} (t)&\ensuremath{\... ...{P}} (t) \end{bmatrix}=\frac{d\ensuremath{\mathbf{P}} (t)}{dt} \end{displaymath} (D.1.9)

Then, not surprisingly, solutions to the CTDRE, (D.0.1), are intimately connected to the properties of the Hamiltonian matrix.

We first note that $\ensuremath{\mathbf{H}} $ has the following reflexive property:


\begin{displaymath} \ensuremath{\mathbf{H}} =- \ensuremath{\mathbf{T}}\ensurema... ...nsuremath{\mathbf{I_n}} & \ensuremath{\mathbf{0}} \end{bmatrix}\end{displaymath} (D.1.10)

where $\ensuremath{\mathbf{I_n}} $ is the identity matrix in $\mathbb{R} ^{n\times n}$.

Recall that a similarity transformation preserves the eigenvalues; thus, the eigenvalues of $\ensuremath{\mathbf{H}} $ are the same as those of $ -\ensuremath{\mathbf{H}} ^T$. On the other hand, the eigenvalues of $\ensuremath{\mathbf{H}} $ and $ \ensuremath{\mathbf{H}} ^T$ must be the same. Hence, the spectral set of $\ensuremath{\mathbf{H}} $ is the union of two sets, $\Lambda_s$ and $\Lambda_u$, such that, if $\lambda\in\Lambda_s$, then $-\lambda\in\Lambda_u$. We assume that $\ensuremath{\mathbf{H}} $ does not contain any eigenvalue on the imaginary axis (note that it suffices, for this to occur, that $(\ensuremath{\mathbf{A}} ,\ensuremath{\mathbf{B}} )$be stabilizable and that the pair $(\ensuremath{\mathbf{A}} ,\ensuremath{\mathbf{\ensuremath{\boldsymbol{\Psi}}^{\frac{1}{2}}}} )$ have no undetectable poles on the stability boundary). In this case, $\Lambda_s$ can be so formed that it contains only the eigenvalues of $\ensuremath{\mathbf{H}} $ that lie in the open LHP. Then, there always exists a nonsingular transformation $\ensuremath{\mathbf{V}}\in\mathbb{R} ^{2n\times 2n}$ such that


\begin{displaymath}[\ensuremath{\mathbf{V}} ]^{-1} \ensuremath{\mathbf{H}}\ensur... ...nsuremath{\mathbf{0}} & \ensuremath{\mathbf{H_u}} \end{bmatrix}\end{displaymath} (D.1.11)

where $\ensuremath{\mathbf{H_s}} $ and $\ensuremath{\mathbf{H_u}} $ are diagonal matrices with eigenvalue sets $\Lambda_s$ and $\Lambda_u$, respectively.

We can use $\ensuremath{\mathbf{V}} $ to transform the matrices $ \ensuremath{\mathbf{M}} (t)$ and $\ensuremath{\mathbf{N}} (t)$, to obtain


\begin{displaymath} \begin{bmatrix} \ensuremath{\mathbf{\tilde{M}}} (t)\\ \ensu... ...th{\mathbf{M}} (t)\\ \ensuremath{\mathbf{N}} (t) \end{bmatrix}\end{displaymath} (D.1.12)

Thus, (D.1.2) can be expressed in the equivalent form:

\begin{displaymath} \frac{d}{dt} \begin{bmatrix} \ensuremath{\mathbf{\tilde{M}... ...e{M}}} (t)\\ \ensuremath{\mathbf{\tilde{N}}} (t) \end{bmatrix}\end{displaymath} (D.1.13)

If we partition $\ensuremath{\mathbf{V}} $ in a form consistent with the matrix Equation (D.1.13), we have that


\begin{displaymath} \ensuremath{\mathbf{V}} = \begin{bmatrix} \ensuremath{\ma... ...th{\mathbf{V_{21}}} &\ensuremath{\mathbf{V_{22}}} \end{bmatrix}\end{displaymath} (D.1.14)

The solution to the CTDRE is then given by the following lemma.

Lemma D.2 A solution for Equation (D.0.1) is given by


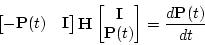
\begin{displaymath} \ensuremath{\mathbf{P}} (t)= \ensuremath{\mathbf{P_1}} (t) [\ensuremath{\mathbf{P_2}} (t)]^{-1} \end{displaymath} (D.1.15)

where

\begin{displaymath} \ensuremath{\mathbf{P_1}} (t)=\ensuremath{\mathbf{V_{21}}} ... ...nsuremath{\mathbf{V_a}} e^{\ensuremath{\mathbf{H_s}} (t_f-t)} \end{displaymath} (D.1.16)


\begin{displaymath} \ensuremath{\mathbf{P_2}} (t)=\bigl[\ensuremath{\mathbf{V_{... ...mathbf{V_a}} e^{\ensuremath{\mathbf{H_s}} (t_f-t)}\bigr]^{-1} \end{displaymath} (D.1.17)


\begin{displaymath} \ensuremath{\mathbf{V_a}}\stackrel{\rm\triangle}{=}-[\ensure... ...(t_f)\left[\ensuremath{\mathbf{\tilde{M}}} (t_f) \right]^{-1} \end{displaymath} (D.1.18)

Proof

From (D.1.12), we have


\begin{displaymath}\ensuremath{\mathbf{M}} (t_f) =\ensuremath{\mathbf{V}} _{11}\... ...remath{\mathbf{V}} _{12}\ensuremath{\mathbf{\tilde{N}}} (t_f) \end{displaymath} (D.1.19)


\begin{displaymath}\ensuremath{\mathbf{N}} (t_f) =\ensuremath{\mathbf{V}} _{21}\... ...thbf{V}} _{22}\ensuremath{\mathbf{\tilde{N}}} (t_f) \nonumber \end{displaymath}  

Hence, from (D.1.3),


\begin{displaymath}\left[ \ensuremath{\mathbf{V}} _{21}\ensuremath{\mathbf{\til... ...lde{N}}} (t_f)\right] ^{-1} =\ensuremath{\boldsymbol{\Psi}} _f \end{displaymath} (D.1.20)

or


\begin{displaymath}\left[ \ensuremath{\mathbf{V}} _{21}+\ensuremath{\mathbf{V}}... ...}} (t_f)]^{-1}\right] ^{-1}=\ensuremath{\boldsymbol{\Psi}} _f \end{displaymath} (D.1.21)

or


\begin{displaymath}\ensuremath{\mathbf{\tilde{N}}} (t_f)[\ensuremath{\mathbf{\ti... ...\ensuremath{\mathbf{V}} _{11}\right] =\ensuremath{\mathbf{V_a}}\end{displaymath} (D.1.22)

Now, from (D.1.10),


\begin{displaymath} \ensuremath{\mathbf{P}} (t)=\ensuremath{\mathbf{N}} (t)[\ensuremath{\mathbf{M}} (t)]^{-1} \end{displaymath} (D.1.23)


\begin{displaymath}=\left[ \ensuremath{\mathbf{V}} _{21}\ensuremath{\mathbf{\til... ...12}\ensuremath{\mathbf{\tilde{N}}} (t)\right] ^{-1} \nonumber \end{displaymath}  


\begin{displaymath}=\left[ \ensuremath{\mathbf{V}} _{21}+\ensuremath{\mathbf{V}}... ...nsuremath{\mathbf{\tilde{M}}} (t)]^{-1}\right] ^{-1} \nonumber \end{displaymath}  

and the solution to (D.1.13) is


\begin{displaymath}\ensuremath{\mathbf{\tilde{M}}} (t_f) =e^{\ensuremath{\mathbf{H}} _s(t_f-t)}\ensuremath{\mathbf{\tilde{M}}} (t) \end{displaymath} (D.1.24)


\begin{displaymath}\ensuremath{\mathbf{\tilde{N}}} (t_f) =e^{\ensuremath{\mathbf{H}} _u(t_f-t)}\ensuremath{\mathbf{\tilde{N}}} (t) \nonumber \end{displaymath}  

Hence,


\begin{displaymath}\ensuremath{\mathbf{\tilde{N}}} (t)[\ensuremath{\mathbf{\tild... ...f{\tilde{M}}} (t_f)]^{-1}e^{\ensuremath{\mathbf{H}} _s(t_f-t)} \end{displaymath} (D.1.25)

Substituting (D.1.25) into (D.1.23) gives the result.

$\Box \Box \Box $